Mathematics Introduction, Direction Cosines and Direction Ratios of a Line And Relation between the direction cosines of a line
Please Wait... While Loading Full Video

Class 12 Chapter 11 - THREE DIMENSIONAL GEOMETRY

Introduction, Direction Cosines and Direction Ratios of a Line And Relation between the direction cosines of a line

Topic Covered

`color{red} ♦` Introduction
`color{red} ♦` Direction Cosines and Direction Ratios of a Line
`color{red} ♦` Relation between the direction cosines of a line
`color{red} ♦` Direction cosines of a line passing through two points

Introduction

● In this chapter, we shall study the direction cosines and direction ratios of a line joining two points and also discuss about the equations of lines and planes in space under different conditions, angle between two lines, two planes, a line and a plane, shortest distance between two skew lines and distance of a point from a plane.

Direction Cosines and Direction Ratios of a Line

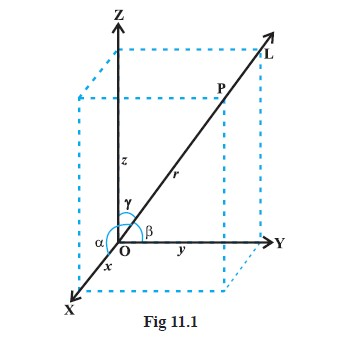
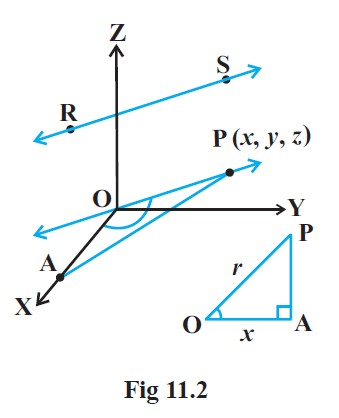
`=>`As we know, If a directed line `L` passing through the origin makes angles `α, β` and `γ` with `x, y` and `z-`axes, respectively, called direction angles, then cosine of these angles, namely, `cos α, cos β` and `cos γ` are called direction cosines of the directed line `L.`

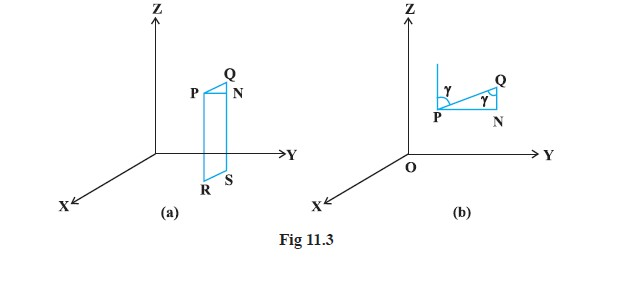
`=>` If we reverse the direction of `L,` then the direction angles are replaced by their supplements,

i.e., `π −α , π − β` and `π − γ.` Thus, the signs of the direction cosines are reversed.


`\color{blue} ✍️` Note that a given line in space can be extended in two opposite directions and so it has two sets of direction cosines. In order to have a unique set of direction cosines for a given line in space, we must take the given line as a directed line. These unique direction cosines are denoted by `l, m` and `n.`

`color {blue} "Key Concepts :"` If the given line in space does not pass through the origin, then, in order to find its direction cosines, we draw a line through the origin and parallel to the given line. Now take one of the directed lines from the origin and find its direction cosines as two parallel line have same set of direction cosines.

`color{blue}{"Direction Ratios : "}` Any three numbers which are proportional to the direction cosines of a line are called the direction ratios of the line. If `l, m, n` are direction cosines and `a, b, c` are direction ratios of a line, then `a = λl, b=λm` and `c = λn,` for any nonzero `λ ∈ R.`

`"Note -"` Some authors also call direction ratios as direction numbers.

● Let `a, b, c` be direction ratios of a line and let `l, m` and `n` be the direction cosines (d.c’s) of the line. Then

`l/a = m/b = n/c = k` (say), k being a constant.

Therefore` l = ak, m = bk, n = ck` ... (1)

But `l^2 + m^2 + n^2 = 1`

Therefore `k^2 (a^2 + b^2 + c^2) = 1`

or ` k = pm (1)/( sqrt (a^2 + b^2 + c^2 ) )`

Hence, from (1), the d.c.’s of the line are

`color{red}{ l = pm a/(sqrt (a^2 + b^2 + c^2 ) ) , m = pm b/(sqrt (a^2 + b^2 + c^2 ) ) , n = pm c/(sqrt (a^2 + b^2 + c^2) )}`

`=>` where, depending on the desired sign of k, either a positive or a negative sign is to be taken for l, m and n.

`=>` For any line, if a, b, c are direction ratios of a line, then `ka, kb, kc; k ≠ 0` is also a set of direction ratios. So, any two sets of direction ratios of a line are also proportional.

`=>` Also, for any line there are infinitely many sets of direction ratios.

Relation between the direction cosines of a line

● Consider a line `RS` with direction cosines `l, m, n.` Through the origin draw a line parallel to the given line and take a point `P(x, y, z)` on this line. From `P` draw a perpendicular `PA` on the x-axis (Fig.).

`=>` Let `OP = r,` Then `cos alpha = (OA)/(OP) =x/r` . This gives `x = lr.`

Similarly, `y = mr` and `z = nr`

Thus `x^2 + y^2 + z^2 = r^2 (l^2 + m^2 + n^2)`

But `x^2 + y^2 + z^2 = r^2`

Hence `color{blue}{l^2 + m^2 + n^2 = 1}`

Direction cosines of a line passing through two points

`=>` Since one and only one line passes through two given points, we can determine the direction cosines of a line passing through the given points `P(x_1, y_1, z_1)` and `Q(x_2, y_2, z_2)` as follows (Fig (a)).

`=>` Let `l, m, n` be the direction cosines of the line `PQ` and let it makes angles `α, β` and `γ` with the `x, y` and `z-`axis, respectively.

`=>` Draw perpendiculars from P and Q to XY-plane to meet at R and S. Draw a perpendicular from P to QS to meet at N. Now, in right angle triangle PNQ, `∠PQN= γ` (Fig (b).

`=>` `cos gamma = (NQ)/(PQ) = (z_2- z_1)/(PQ)`

Similarly `cos α = (x_2- x_1)/(PQ)` and `cos beta = (y_2-y_1)/(PQ)`

`\color{green} ✍️` Hence, the direction cosines of the line segment joining the points `P(x_1, y_1, z_1)` and `Q(x_2, y_2, z_2)` are

`color{green}{(x_2- x_1)/(PQ) , (y_2- y_1)/(PQ) , (z_2 -z_1)/(PQ)}`

where `PQ= sqrt ( (x_2 -x_1)^2 + (y_2 - y_1 )^2 +( z_2 -z_1)^2)`

`color{red} "Key Point"` - The direction ratios of the line segment joining `P(x_1, y_1, z_1)` and `Q(x_2, y_2, z_2)` may be taken as

`color{blue}{x_2 – x_1, y_2 – y_1, z_2 – z_1` or `x_1 – x_2, y_1 – y_2, z_1 – z_2}`




Q 3187478387

If a line makes angle 90°, 60° and 30° with the positive direction of x, y and
z-axis respectively, find its direction cosines.
Class 12 Chapter 11 Example 1
Solution:

Let the d.c . 's of the lines be l , m, n. Then `l = cos 90^o = 0, m = cos 60^o = 1/2` ,

`n = cos 30^o = (sqrt 3)/2`
Q 3117478389

If a line has direction ratios 2, – 1, – 2, determine its direction cosines.
Class 12 Chapter 11 Example 2
Solution:

Direction cosines are

`2/(sqrt (2^2 + (-1)^2 + (-2)^2 ) ) , (-1)/(sqrt (2^2 + (-1)^2 + (-2)^2) ) , (-2)/(sqrt ( 2^2 + (-1)^2 + (-2)^2) )`

or `2/3 , (-1)/3 , (-2)/3`
Q 3127578481

Find the direction cosines of the line passing through the two points
(– 2, 4, – 5) and (1, 2, 3).
Class 12 Chapter 11 Example 3
Solution:

We know the direction cosines of the line passing through two points
`P(x_1, y_1, z_1)` and `Q(x_2, y_2, z_2)` are given by

`(x_2 - x_1)/(PQ) , (y_2 - y_1)/(PQ) , (z_2 -z_1)/(PQ)`

where `PQ= sqrt ( (x_2- x_1 )^2 + ( y_2 - y_1)^2 + ( z_2 -z_1)^2 )`

Here P is (– 2, 4, – 5) and Q is (1, 2, 3).

So `PQ = sqrt ( (1- (-2) )^2 + (2-4)^2 + (3 - (-5) )^2 ) = sqrt (77)`

Thus, the direction cosines of the line joining two points is

`3/(sqrt 77) , (-2)/(sqrt 77) , 8/(sqrt 77)`
Q 3157578484

Find the direction cosines of x, y and z-axis.
Class 12 Chapter 11 Example 4
Solution:

The x-axis makes angles 0°, 90° and 90° respectively with x, y and z-axis.
Therefore, the direction cosines of x-axis are cos 0°, cos 90°, cos 90° i.e., 1,0,0.
Similarly, direction cosines of y-axis and z-axis are 0, 1, 0 and 0, 0, 1 respectively.
Q 3167578485

Show that the points A (2, 3, – 4), B (1, – 2, 3) and C (3, 8, – 11) are
collinear.
Class 12 Chapter 11 Example 5
Solution:

Direction ratios of line joining A and B are
1 – 2, – 2 – 3, 3 + 4 i.e., – 1, – 5, 7.
The direction ratios of line joining B and C are
3 –1, 8 + 2, – 11 – 3, i.e., 2, 10, – 14.
It is clear that direction ratios of AB and BC are proportional, hence, AB is parallel
to BC. But point B is common to both AB and BC. Therefore, A, B, C are
collinear points.
 
SiteLock